What is Problem-Based Learning?
By Tim Woodbine
Maths Coach at New Tech Network Australia
What is PrBL and how does it work for Maths?
PrBL is the abbreviation for Problem-Based Learning and is the recommended pedagogy for mathematics within the New Tech Network. PrBL is a pedagogy in which the teacher starts the lesson with a real world problem, ideally authentic to a student's life.
After the problem launch the teacher does not teach, they in fact let the students grapple with the problem for approximately 15 minutes.
The only tool the teacher is armed with is guided questions. A guided question is where a teacher uses questioning to promote thought in the right direction. Of course a teacher cannot expect students to be able to solve a worthwhile problem within 15 minutes, hence a student-centred scaffolding activity is then required. A scaffolding activity should lead to that “A- HA” moment, in which a student will often state “I get it now”. Once the student has unearthed the necessary skill they are able to go back to the original problem and hopefully solve it. When a student has gained the expertise to master a problem they need to spend time committing this new strength to their long term memory bank, therefore some deliberate practice is required. A problem should only take a lesson or two. Key components to PrBL is the use of collaboration and fostering feedback from their peers, we will expand on these concepts later in the article.
What is an example of a PrBL Maths problem?
Please watch the entry event video below:
After watching the video, the question is ‘Will the squirrel win the race?’
The timetable in our schools will vary vastly, consequently, teachers should anticipate what students already know about the problem and what they need to know. If time is an issue then you may choose to provide most of the information. If you have plenty of time, then you may wait for students to clearly state what is the key information required to solve the problem. Once students can articulate what is essential to solve the problem, they will be given the appropriate information.
From the problem example above, here are the anticipated Knows and Need to Knows:
Once you have anticipated the Need to Knows, you may wish to provide certain information. Like the information below:
Normal guy got a 3.43 second head start.
The normal guy ran for 40 feet in 2 seconds.
The squirrel ran for 56 feet in 2 seconds.
What happens next in Problem-Based Learning?
At times a teacher will need to progress the problem. For example with the problem above, I would expect approximately 50% of students to need assistance with Pythagoras Theorem. It is at this point in PrBL we run workshops to give students the necessary skills to complete the problem. We may also provide the students with a scaffolding activity or complete a gallery walk, where students view other students' work. All these tasks are focused on giving students the required skills to complete the problem. Once students have these essential skills they finish off the problem by sharing out their work, or the teacher debriefing the class or finally by revisiting the Need to Knows to ensure all students have gained the appropriate skills.
PrBL is not a new pedagogy.
Problem-Based Learning has been around for decades. In the video below from 2010 Dan Meyer emphasises the need for mathematics classrooms to have a makeover and further illustrates what a quality Maths problem looks like.
Today's math curriculum is teaching students to expect -- and excel at -- paint-by-numbers classwork, robbing kids of a skill more important than solving problems.
What are the essential components of Problem-Based Learning for Maths?
Each problem has essential components. The essentials of PrBL include:
Staff need to identify and anticipate the knows and need to knows before they release the problem.
Visuals are essential for problems.
Look for connected mathematics syllabus outcomes.
The PrBL process doesn’t go from 0% to 100%. It is a slow process , so aim for staff to do 7-12 problems in the first year.
What do teachers do when they are not teaching via Problem-Based Learning? Smaller activities that promote math inquiry and discourse.
A key aspect of PrBL is the notion that mathematics classrooms have the order mistaken
If we were teaching Pythagoras Theorem in most Australian mathematics classrooms, I would expect the teacher to start the lesson by introducing the formula of Pythagoras Theorem. The teacher would then show the students how to use the formula, and the students would copy the example down. Effectively we give students Pythagoras Theorem before creating a need to learn Pythagoras Theorem. I feel the following simile describes mathematics classrooms in Australia well, ‘we give students the panadol before causing the headache.’
We need to create the headache before the panadol is provided.
In regards to Pythagoras Theorem, we need to create a situation where students need to find the length of the hypotenuse of a right angled triangle. As students struggle to find a way to evaluate how to find the length of a hypotenuse, we can then provide them with the panadol, i.e Pythagoras Theorem.
Problem-Based Learning and Project-Based Learning: Cut From the Same Cloth But With Some Notable Differences
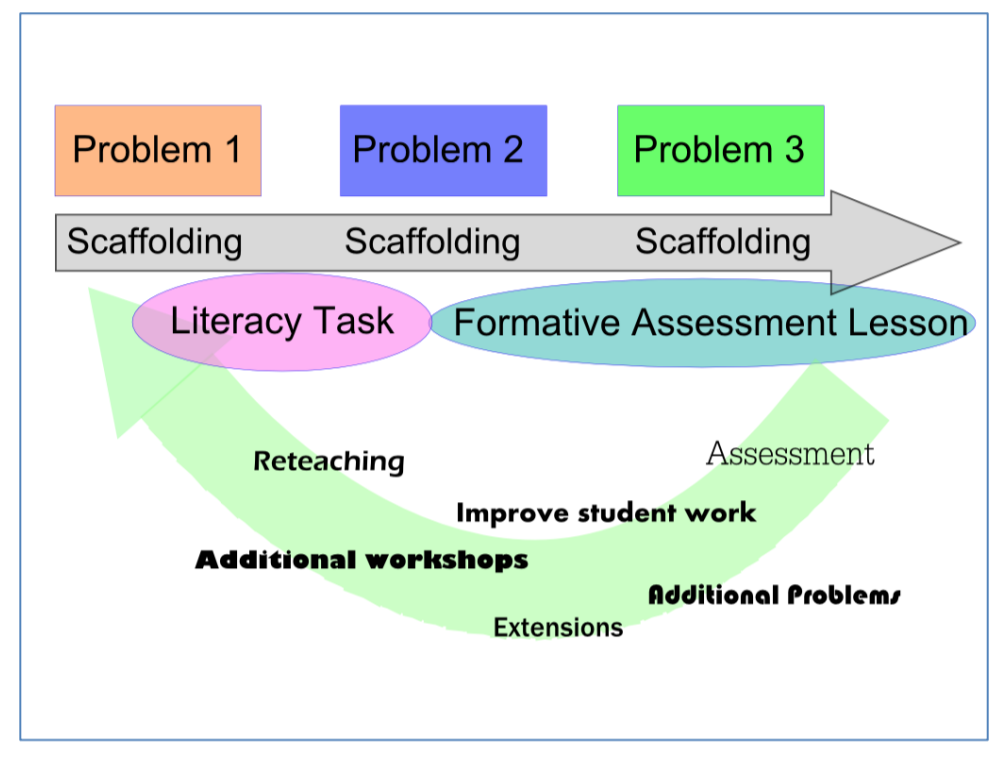
Throughout my time teaching PrBL, teachers often confuse it with Project Based Learning (PBL). There are many misconceptions about PrBL being the same as PBL. In PrBL we do not require an end product. The length of a problem is a lesson or two. A mathematics PrBL unit will be divided into many smaller problems and not one overarching project. The diagrams below show the differences between a problem and project unit, as well as specifically how a problem unit appears.
When starting out with PrBL, a common mistake is to get lost in the context of the project
After watching the Year 7 STEM video below consider this: when the students went home, what did they tell their parents they learned that day? Would the students state the context or the content?
After reflecting on my practices in PrBL, I now believe my early attempts on problems were very context focused. All our problems were focused on an engaging context and when students would go home, parents would always ask “What did you learn today?” Nearly every time students would reply with the context (in this case firing bottle rockets). The students would not see the focus was the content (i.e. the parabolas followed by each of the rockets). In PrBL the focus is always the mathematics and not the context. At times I have presented students with 2 problems on the same content, but with differing contexts. I will then ask students to state what the content is within the problems. Students need to see the content as the main focus.
How do I get started with Problem-Based Learning in my Maths Classroom?
As teachers start to create their first PrBL unit, they often ask what is the structure of a problem. As a starting point a problem should contain an entry event (the problem launch), a scaffolding activity and some deliberate practice.
After implementing PrBL in multiple schools I understand a need to see the physical look of a PrBL classroom. The video below shows how students are seated and how they interact in a PrBL setting. As demonstrated in the video PrBL promotes feedback from their peers, which in turn fuels learning. PrBL leads to students not waiting long at all to receive feedback on their work, which results in improved academic achievement in Maths. Problem-Based Learning Maths Classrooms foster communication, collaboration, critical thinking and mathematical discourse, key skills which students need for success at Year 12 and beyond their High School Graduation.
Are you ready to learn more? Why not join us for a PrBL for Maths 101 or 201 course. Details below: